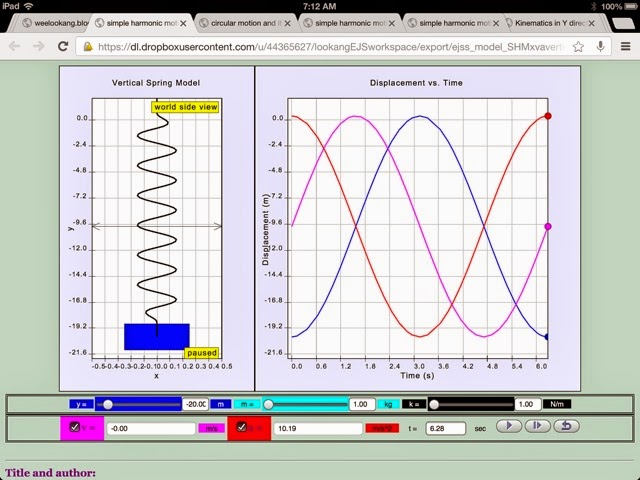
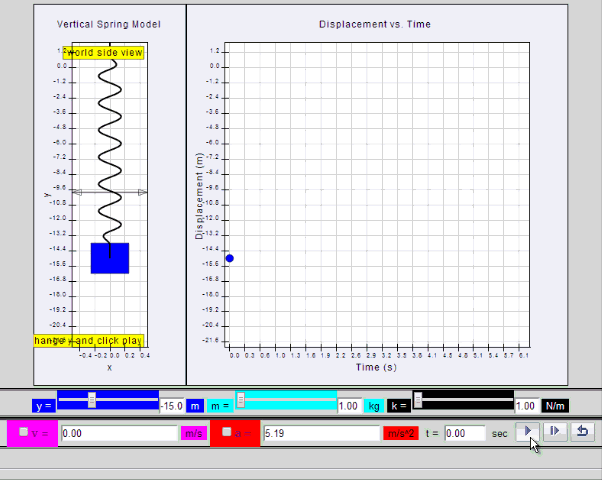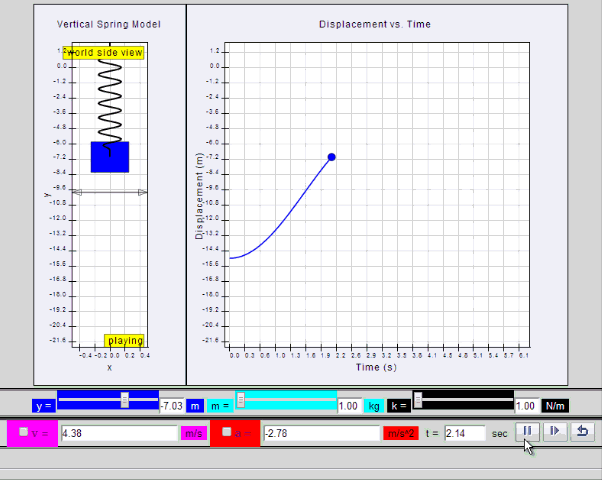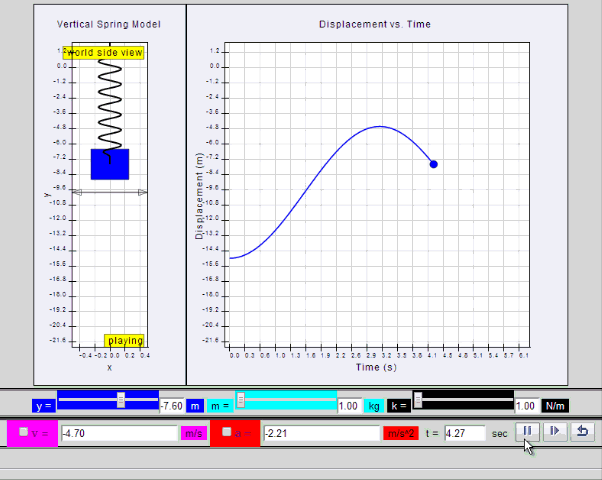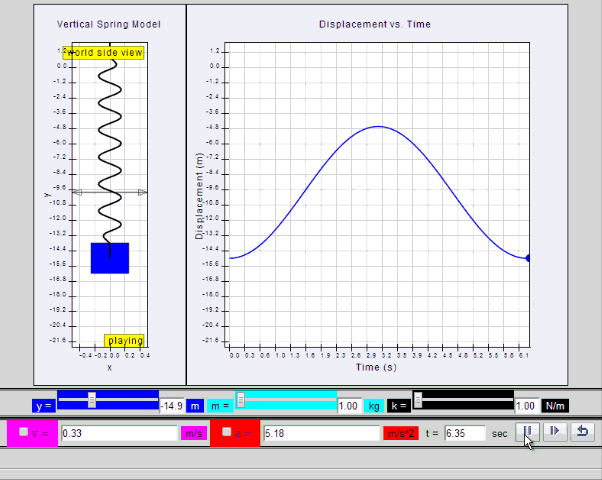
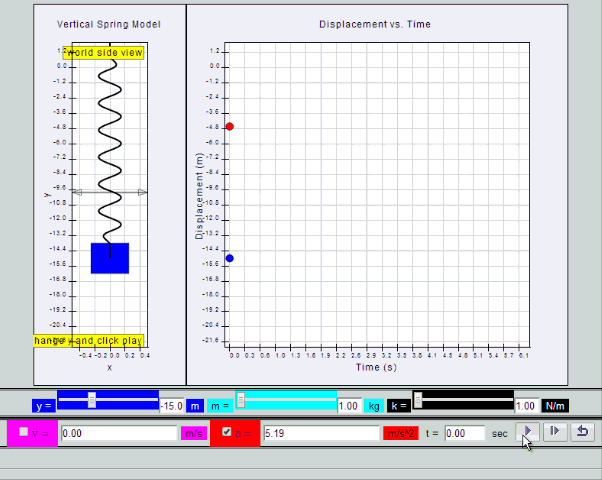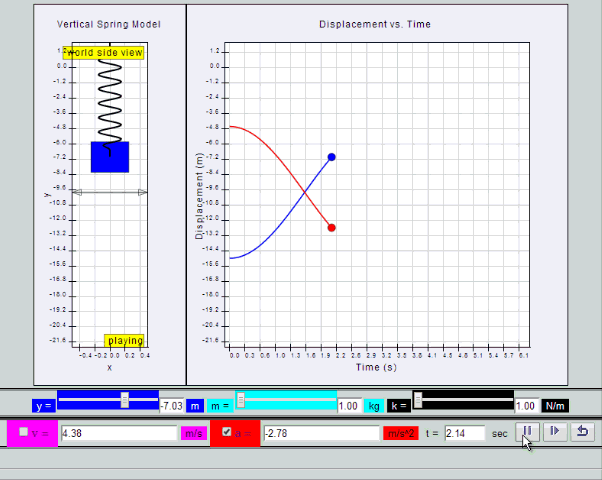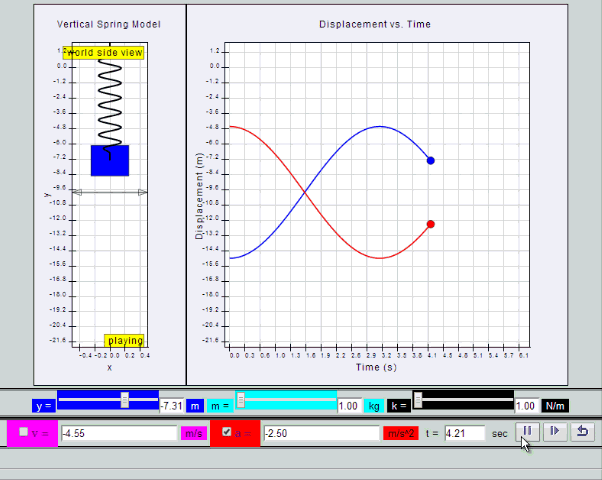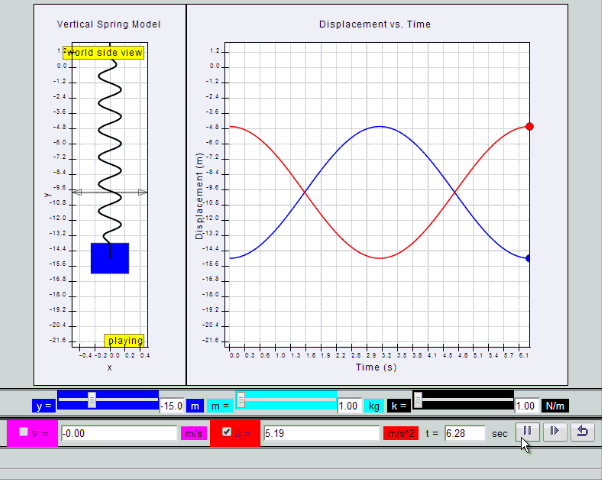
EJSS simple harmonic motion vertical spring mass model with y vs t, v vs t and a vs t graph
based on models and ideas by
- lookang http://weelookang.blogspot.sg/2014/02/ejss-shm-model-with-vs-x-and-v-vs-x.html
- lookang http://weelookang.blogspot.sg/2010/06/ejs-open-source-simple-harmonic-motion.html?q=SHM
- lookang http://weelookang.blogspot.sg/2013/02/ejs-open-source-vertical-spring-mass.html?q=vertical+spring
- Wolfgang Christian and Francisco Esquembre http://www.opensourcephysics.org/items/detail.cfm?ID=13103
Assumption:
Motion approximates SHM when the spring does not exceed limit of proportionality during oscillations.The equations that model the motion of the spring mass system are:
where F is
the restoring elastic force exerted by the spring (in SI
units: N),
k is the spring
constant (N·m−1),
and y is the displacement
from the equilibrium position (in m).
δyδt=vy
δvyδt=−km(y−l)−g
where the terms
−km(y−l) represents the restoring
force component as a result of the spring extending and
compressing.
−g represents the gravity
force component as a result of Earth's pull.
Once the mass is displaced from its equilibrium position, it
experiences a net restoring force. As a result, it accelerates
and starts going back to the equilibrium position. When the
mass moves closer to the equilibrium position, the restoring
force decreases. At the equilibrium position, the net
restoring force vanishes. However, at y = 0, the mass has momentum
because of the impulse
that the restoring force has imparted. Therefore, the mass
continues past the equilibrium position, compressing the
spring. A net restoring force then tends to slow it down,
until its velocity
reaches zero, whereby it will attempt to reach equilibrium
position again.
v=yoωsin(ωt)=yoωcos(ωt−π2)
therefore , in general:
y=yosin(ωt−ϕ)
ϕ=π/2
for a starting position of y=−yo
ϕ=−π/2
for a starting position of y=yo
v=yoωcos(ωt−ϕ)
a=−yoω2sin(ωt−ϕ)
Thus, this model assumes
where the terms
What is SHM?
Simple harmonic motion is typified by the motion of a mass on a spring when it is subject to the linear elastic restoring force given by Hooke's Law. The motion is sinusoidal in time and demonstrates a single resonant frequency. In order for simple harmonic motion to take place, the net force of the object at the end of the pendulum must be proportional to the displacement. In other words, oscillations are periodic variations in the value of a physical quantity about a central or equilibrium value.If motion starts at the equilibrium position and starts to move to the positive direction solutions to the defining equation are:
not possible, as it is in equilibrium.
If the motion starts to the negative amplitude position:
therefore , in general:
where